'

Search results

Found 1093 matches
Klein bagel (4-D non-intersecting parameterization x- coordinate)

n mathematics, the Klein bottle is an example of a non-orientable surface, informally, it is a surface (a two-dimensional manifold) in which notions of ... more

Nose cone ogive shape (radius y at any point x)

Nose cone section of any vehicle or body meant to travel through a compressible fluid medium (such as a rocket or aircraft, missile or bullet) has several ... more

Nose cone Spherically blunted tangent ogive( X- coordinate of the tangency point )

The nose cone section of any vehicle or body meant to travel through a compressible fluid medium (such as a rocket or aircraft, missile or bullet) is ... more

Nose cap Spherically blunted tangent ogive shape ( X-coordinate of the center)

The tangent ogive shape nose-cap is the most familiar in hobby rocketry. The profile of this shape is formed by a segment of a circle such that the rocket ... more

Nose cone Spherically blunted tangent ogive( Y- coordinate of the tangency point )

The nose cone section of any vehicle or body meant to travel through a compressible fluid medium (such as a rocket or aircraft, missile or bullet) is ... more

Klein bagel (4-D non-intersecting parameterization z-coordinate)

In mathematics, the Klein bottle is an example of a non-orientable surface, informally, it is a surface (a two-dimensional manifold) in which notions of ... more

Klein bagel (4-D non-intersecting parameterization w-coordinate)

In mathematics, the Klein bottle is an example of a non-orientable surface, informally, it is a surface (a two-dimensional manifold) in which notions of ... more

Rhodonea curve

In mathematics, a rose or rhodonea curve is a sinusoid plotted in polar coordinates. the polar coordinate system is a two-dimensional coordinate system in ... more

Area moments of inertia for a filled quarter circle with respect to a horizontal or vertical axis through the centroid

The second moment of area, also known as moment of inertia of plane area, area moment of inertia, polar moment of area or second area moment, is a ... more

Worksheet 334

In a video game design, a map shows the location of other characters relative to the player, who is situated at the origin, and the direction they are facing. A character currently shows on the map at coordinates (-3, 5). If the player rotates counterclockwise by 20 degrees, then the objects in the map will correspondingly rotate 20 degrees clockwise. Find the new coordinates of the character.

To rotate the position of the character, we can imagine it as a point on a circle, and we will change the angle of the point by 20 degrees. To do so, we first need to find the radius of this circle and the original angle.

Drawing a right triangle inside the circle, we can find the radius using the Pythagorean Theorem:

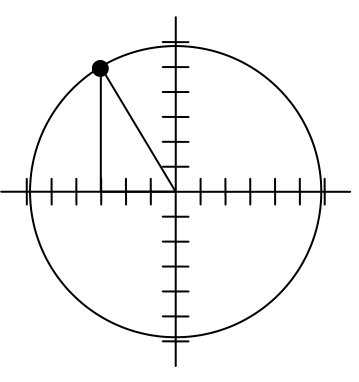
Pythagorean theorem (right triangle)

To find the angle, we need to decide first if we are going to find the acute angle of the triangle, the reference angle, or if we are going to find the angle measured in standard position. While either approach will work, in this case we will do the latter. By applying the cosine function and using our given information we get

Cosine function
Subtraction

While there are two angles that have this cosine value, the angle of 120.964 degrees is in the second quadrant as desired, so it is the angle we were looking for.

Rotating the point clockwise by 20 degrees, the angle of the point will decrease to 100.964 degrees. We can then evaluate the coordinates of the rotated point

For x axis:

Cosine function

For y axis:

Sine function

The coordinates of the character on the rotated map will be (-1.109, 5.725)

Reference : PreCalculus: An Investigation of Functions,Edition 1.4 © 2014 David Lippman and Melonie Rasmussen
http://www.opentextbookstore.com/precalc/
Creative Commons License : http://creativecommons.org/licenses/by-sa/3.0/us/

...can't find what you're looking for?

Create a new formula