Moment of Inertia - Point mass
Description
Moment of inertia is the mass property of a rigid body that determines the torque needed for a desired angular acceleration about an axis of rotation. Moment of inertia depends on the shape of the body and may be different around different axes of rotation. A larger moment of inertia around a given axis requires more torque to increase the rotation, or to stop the rotation, of a body about that axis. the mass moment of inertia, usually denoted by I, measures the extent to which an object resists rotational acceleration about an axis, and is the rotational analogue to mass. For simple objects with geometric symmetry, one can often determine the moment of inertia in an exact closed-form expression. A point mass does not have a moment of inertia around its own axis, but using the parallel axis theorem a moment of inertia around a distant axis of rotation is achieved. Point mass m at a distance r from the axis of rotation has moment of inertia depended on the mass and the distance from the axis of rotation. 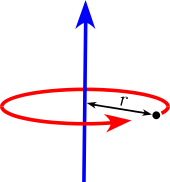
Variables
| I | Moment of Inertia or a point mass (kg*m2) |
| m | Mass (kg) |
| r | Distance from axis of rotation (m) |