Area of a Disc (integral)
Description
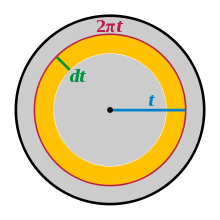
Calculates the area of a disc of radius r or the area enclosed in a circle of radius r. Partitioning the disk into thin concentric rings, like the layers of an onion, the area of the disk can be calculated by the method of shell integration in two dimensions. For an infinitesimally thin ring of the “onion” the accumulated area is the circumferential length of the ring times its infinitesimal width.
This gives an elementary integral for a disk of radius r
 Related formulas
Related formulas
Variables
| Ad | Area of disc (dimensionless) |
| r | Radius of disc (dimensionless) |
| x | Infinitely thin slice (onion ring) (dimensionless) |
| π | pi |