Lately, some selective hearing devices have surfaced. First these Active Listening Ear-buds and lately this Noise Canceling helmet. Both these systems are letting you hear whatever you want. In the first case, you can reduce the volume of a crying baby, or amplify the music in a concert the way you want. The helmet’s manufacturers doesn’t really care about crying babies. They want the biker to be undisrupted on his motorcycle and not get tired by the constant buzzing that is generated by the pressurized air forcing its way through the helmet’s ventilation channels. But it is vital for the rider to be able to hear everything else happens to the road he is driving. That is why that helmet eliminates only the unwanted buzzing.
I guess that this technology is based on sound waves and wavelength.

Wavelength is the distance between repeating units of a propagating wave of a given frequency.
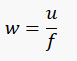
It depends on the medium (vacuum, air, or water) that a wave travels through. Sound is a vibration that propagates as a typically audible mechanical wave of pressure and displacement, through a medium such as air or water.
For sound waves in air, the speed of sound is 343 m/s (at room temperature and atmospheric pressure. The wavelengths of sound frequencies audible to the human ear (20 Hz–20 kHz) are thus between approximately 17 m and 17 mm, respectively.
The Sound Wavelength is given by the equation on the left (and up), where w is the wavelength in meters, u the wave Velocity in (m/s) and F the wave frequency (Hz).

In the case of longitudinal harmonic sound waves, the wavelength can be calculated by the distance the point has traveled from the wave’s source, the time elapsed, the amplitude of the oscillation, the speed of the wave and the angular frequency of the wave. For sound waves, the amplitude of the wave is the difference between the pressure of the undisturbed air and the maximum pressure caused by the wave.
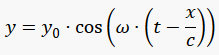
The formula that gives their wavelength is the one on the left. y0 is the amplitude of the oscillation (m), ω the angular frequency (radians/sec), t the time elapsed (sec), x the distance the point has traveled from the wave’s source (m) and c is the speed of the wave (m/s).

Go ahead, check this equation and search whatever else you want to solve. You will find some help in our fxSolver video.
Also remember to follow or interact with us in our social media pages. You will find links below.