The hovercraft was invented by Christopher Cockerell in 1956. The theory behind one of the most successful inventions of the 20th century, the Hovercraft, was originally tested in 1955 using an empty KiteKat cat food tin inside a coffee tin, an industrial air blower and a pair of kitchen scales. Sir Christopher Cockerell developed the first practical hovercraft designs, these led to the first hovercraft to be produced commercially, the SRN1.
Christopher Cockerell’s idea was to build a vehicle that would move over the water’s surface, floating on a layer of air. This would reduce friction between the water and vehicle. To test his hypothesis, he put one a smaller can inside a larger can and used a hairdryer to blow air into them. The downward thrust produced was greater when one can was inside the other rather than air just being blown into one can.
Early interest in hovercraft enjoyed a peak in the early 1960’s as everyone jumped to take advantage of this amazing vehicle. However, by the end of the decade only the British had produced a range of feasible and practical craft.
The problems inherent of the air cushion vehicle, such as Cockerell and others had foreseen, regarding steering control, noise, salt and skirt erosion, caused many countries to abandon their hovercraft development programs in favor of other, more established multi-function vehicles or to use different vehicles specialized in each terrain or function.
Since the 1970’s however, and especially over the last decade, a renewed interest in the hovercraft as (passenger) transport, military transport and weapons and exploratory vehicle has taken ground, solving many of these problems in their development. Lately, even a hover bike made an appearance and US army rushed to bring it in. Also, we should always remember what Mitch Buchannon taught us, that a hovercraft is faster than a fake life guard .

The basic hover principal is expressed in this Thrust to power equation. Thrust is a reaction force described quantitatively by Newton’s second and third laws.
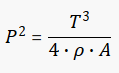
The power needed to generate thrust and the force of the thrust can be related in a non-linear way. Note that these calculations are only valid for when the incoming air is accelerated from a standstill (for example when… hovering).
P: power needed to generate thrust (W), T: thrust generated (force) (N), ρ: density of the fluid (kg/m3), Α: area of the cross section of the propelled volume of fluid (m2)

As always, you can try using fxSolver, with the Thrust to power equation in our database.
Please, remember to follow our social media pages, by hitting the buttons below and watch the fxSolver video as well!