Top 10 Ballistics equations

Ballistics is the science of mechanics that deals with the launching, flight, behavior, and effects of projectiles, especially bullets, gravity bombs, rockets even baseballs. Here are the 10 equations you should have in mind if you are working on ballistics.

1. Escape Velocity

Escape velocity is the speed at which the kinetic energy plus the gravitational potential energy of an object is zero. It is the speed needed to “break free” from a gravitational field without further propulsion. For a spherically symmetric massive body, the escape velocity at a given distance can be calculated by the mass of the massive body, and the distance from the center of gravity. ( Atmospheric friction (air drag) is not taken into account).

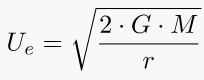
2. Trajectory Height

Trajectory Height A trajectory or flight path is the path that a moving object follows through space as a function of time. A trajectory can be described mathematically either by the geometry of the path, or as the position of the object over time. The maximum height reached by a projectile following a ballistic (parabolic) course is depended on the initial velocity and the initial angle.

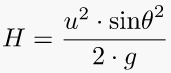
3. Trajectory Range

Trajectory Range Height's best buddy is Range. The range of a projectile following a ballistic trajectory is the greatest distance the object travels along the x-axis. The x-axis is parallel to the ground and the y axis perpendicular to it ( parallel to the gravitational field lines ) and is depended on the initial velocity and the initial angle.

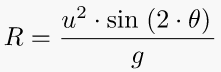
4. Range of a projectile

Assuming that Earth is flat and with a uniform gravity field and no air resistance, the range of a projectile launched with specific initial conditions will have a predictable range and it is calculated by this equation. That applies for ranges which are small compared to the size of the Earth.

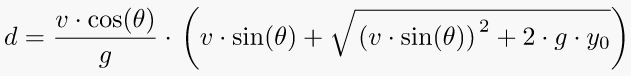
5. Angle of elevation

Angle of Elevation For a required range the angle of projectile launch ( the angle at which a projectile must be launched in order to go a distance , given the initial velocity) is related to the range and the initial velocity. Thank god we have an equation solver here, so the arcsin calculation is easier than ever.

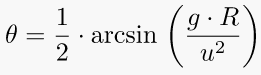
6. Angle required to hit for a projectile following a ballistic trajectory (coordinate (x,y))

Angle Required to hit for a projectile The ballistic trajectory of a projectile is the path that a thrown or launched projectile will take under the action of gravity, neglecting all other forces, such as friction from air resistance, without propulsion.
To hit a target at range x and altitude y when fired from (0,0) and with initial speed u the required angle of launch is depended on the initial velocity.

7. Ballistic Coefficient – using body length

Ballistic Coefficient Body length In ballistics, the ballistic coefficient (BC) of a body is a measure of its ability to overcome air resistance in flight. It is inversely proportional to the negative acceleration — a high number indicates a low negative acceleration. This is roughly the same as saying that the projectile in question possesses low drag, although some meaning is lost in the generalization. BC is a function of mass, diameter, and drag coefficient.

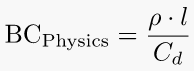
8. Ballistic Coefficient – using corss-sectional area

Ballistic Coefficient Cross sectional area Well, it is pretty much the same principle as number 7, but we need other variables to calculate this type of ballistic coefficient, such as mass, drag coefficient, and the cross-sectional area, instead of density and body length which are used in the 7th equation of our list.

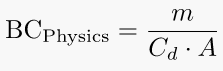
9. Velocity at a distance x (for object following a ballistic trajectory)

Velocity at a distance X Well, the magnitude of the velocity of the projectile at distance x is depended on the initial velocity and the angle at which the projectile is launched. It is given by this equation which is not as difficult as it looks.

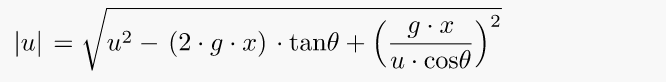
10. Time of flight for a projectile following a ballistic trajectory

Time of flight for a projectile The time of flight is the time it takes for the projectile to finish its trajectory and can be calculated by the angle at which the projectile is launched, the velocity at which the projectile is launched and the initial height of the projectile.

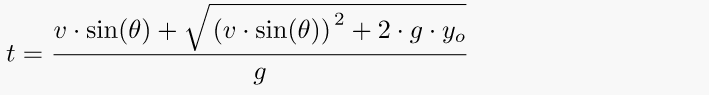
You can try to search whatever you want just by clicking on the “Browse Formulas” button on the top left of your screen.

If you need any help, you will find some in our fxSolver video.
Also remember to follow or interact with us in our social media pages. You will find links below.